emergency Landing in Sint Maarten After Smoke Detected Mid-Flight
Table of Contents
January 19, 2025 – 1 minute read
An Air antilles aircraft flying from Saint-Barthélemy to Pointe-à-Pitre in Guadeloupe was forced to make an unexpected landing at Princess Juliana Airport in Sint Maarten earlier today. The pilots noticed smoke in the cockpit shortly after takeoff from Rémy de Haenen Airport on Saint-barthélemy. In response, they activated the emergency code Squawk 7700, a worldwide distress signal, which promptly alerted emergency teams.
The Twin Otter DHC-6 plane touched down safely at 10:14 AM local time. All passengers and crew members emerged from the incident without injury. arrangements have been made to transport travelers to Pointe-à-Pitre using alternate flights.
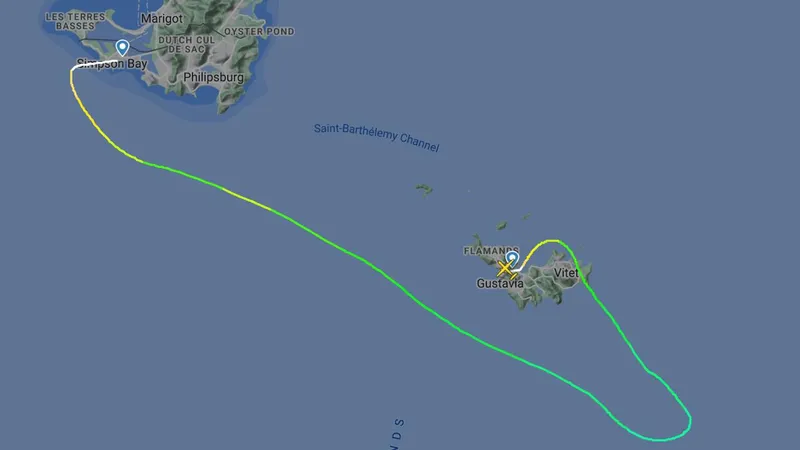emergency landing” class=”wp-image-107214″ srcset=”https://curacao.nu/wp-content/uploads/sites/5/2025/01/flightradar.webp 800w, https://curacao.nu/wp-content/uploads/sites/5/2025/01/flightradar-320×181.webp 320w” sizes=”(max-width: 800px) 100vw, 800px” />Air Antilles clarified that the aircraft was leased from Swiss carrier Zimex and is uniquely equipped to handle short runways, a necessity for flights to Saint-Barthélemy. Following the incident, the airline has suspended all operations to and from the island temporarily. A Zimex technician has been dispatched to Sint maarten to investigate the source of the smoke.
Fire trucks were on standby during the landing, though their assistance was not required. Air Antilles’ Director General, samuel Braconnier, emphasized that safety is the airline’s top priority. “The incident was managed with utmost professionalism,” he stated.
What are some examples of how the empty set is used in mathematical proofs?
The ∅ symbol in mathematics represents the “Empty Set,” wich is a basic concept in set theory. An empty set is a set that contains no elements, making it unique and significant in various mathematical contexts.
Key Points About the Empty Set:
- Definition: The empty set is denoted by the symbol ∅ and is defined as a set with no members. It is sometimes represented as
{}.
- Properties:
– Subset of Every Set: The empty set is a subset of every set, including itself. This means that for any set ( A ), ( emptyset subseteq A ).
– Cardinality: The cardinality (number of elements) of the empty set is zero.
– Unique Identity: There is only one empty set, as all sets with no elements are considered identical.
- Applications:
– Foundational Role: The empty set serves as the foundation for constructing other sets in set theory.
– Mathematical Proofs: It is indeed frequently enough used in proofs to demonstrate properties of sets or functions.
– Operations: The empty set is involved in operations like union, intersection, and Cartesian products.
Example:
- If ( A = {1, 2, 3} ) and ( B = emptyset ), then ( A cup B = A ) and ( A cap B = emptyset ).
Understanding the empty set is crucial for grasping more advanced mathematical concepts, as it plays a pivotal role in the structure of set theory and beyond.For further details,you can explore the provided source.
