Updated:
Keep
The end of the world is not expected for 2342 (perhaps sooner).
There are many authors of recreational mathematics (and science in general) who have devised questions, exercises and problems, framing them and giving them life within some classic work or imitating them. In 1907, for example, the British Henry Ernest Dudeney (along with the American Samuel Loyd, possibly the most notable inventors of problems and puzzles in history), publishes ‘The Canterbury Puzzles’, a set of situations and mathematical enigmas in the mouths of the group of pilgrims who star in the famous work 14th century poet Geoffrey Chaucer. Or ‘The man who calculated’, from the fictional Malba Hold, with more than 54 editions since its first edition in 1938.
With this inspiration I wrote the story of the end of the world framed in the Alhambra that appeared in this section a fortnight ago, placing it in parallel terms to the magnificent ‘Tales of the Alhambra’. To my surprise, there were those who assumed the story as real, although it was well indicated that it was a fiction with the purpose of offering the reader a mathematical puzzle to solve. I appreciate the implicit compliment of knowing that, once my teaching career is over, I may be able to dedicate myself to fantastic narrative.
With that cleared up, let’s go back through the street of the image to the place and solve the question that was important for me, mathematics.
In my imagined story, I found on the walls of the palace a mysterious succession of numbers: 1492, 1898, 1936 and 2342. Beneath them, almost indistinguishable, appeared other numbers. The clearest is 777. They are all years of unfortunate memories for the Spanish, would you suppose that something bad is going to happen in our country in 2342? Leaving aside the imaginative interpretations of the meaning of the years of the expression (chosen on purpose for that purpose), the aim was to show that

is divisible by 777 for any nonnegative integer exponent n. And by the way, find out if there are more numbers apart from 777 with this property.
Everyone in their school years has studied polynomials, and their basic operations. Polynomials are a very interesting mathematical concept because working with them in these operations is very simple (I am not just referring to adding, subtracting, multiplying and dividing; substituting numerical values in them or calculating their derivatives and primitives, it is one of the few things that any student would want to be entered in an exam because he would have an A for sure). That is why polynomials are used as a first approximant to more complicated functions.
Among the most interesting aspects of polynomials (and one of the most complicated), is their factorization, that is, expressing them as a product or quotient of other polynomials of lower degree. The smaller the degree of a polynomial (the higher exponent value that appears), the more manageable it is, obviously. Among the factorizations that we have studied in school, is the famous “difference of squares”, which we learned by constantly repeating it to us that it is equal to “sum times difference”. Algebraically:
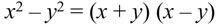
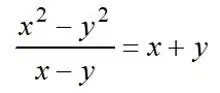
Note that this equality can also be expressed as follows:
If the numbers x and y were integers, that indicates that the quotient of the first member always turns out to be an integer, and equal to x + y. That is, it tells us, for example, that the number
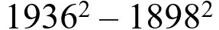
is divisible by 1936 – 1898 = 38, and furthermore we do not need to divide it, because according to the previous expressions, that quotient is 1936 + 1898 = 3834 (those who have a little stinky math, or directly forgotten, can check it : 38 x 3834 = 145692, which is equal to 1936^2 – 1898^2). The wonderful thing regarding the formula is that this is true regardless of the values that we put in x and y. I hope that so far, everything has been understood.
And what regarding exponent 3? Well, there is another formula that is similar, different, but similar (let’s see if the reader discovers that similarity before we describe it):
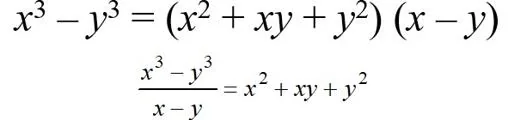
Note that the important idea for our puzzle is that the quotient of the fraction on the first member is an integer (the expression on the second member), as long as x, y are integers.
And sure enough, there are similar formulas for exponent 4, 5, 6, and so on. The expression that generalizes all those exponents, however high they may be, is described by mathematicians as raised to the power n (because n can take any value). That expression is
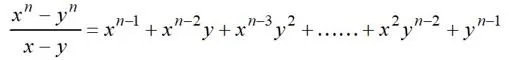
Although it is not very difficult to remember that expression (look at the exponents of each addend: they always add n – 1: first only the x; then we multiply a y, and therefore the x has an exponent of n – 2; then we put y squared, and therefore the x has an exponent n – 3; and so on until the x’s run out, leaving only the y raised to n –1), that IS NOT THE IMPORTANT THING for our enigma. The important thing is that the result of dividing the fraction is a whole number, and therefore x – y divides exactly x^n – y^n.
Let us recall once more the ‘mysterious’ inscription:
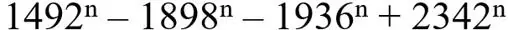
‘Casually’ (yes, it goes with seconds),

I have put them with colors so that they can observe that the four numbers verify that, two by two as indicated, they give the same difference. That means that both 406 and 444 divide (according to the polynomial expression explained before) both addends, that is:
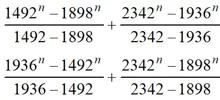
They are integer amounts. Therefore, the entire expression is divisible by 2 × 406 × 444 = 360528 = 2^4 × 3 × 7 × 29 × 37, and thus by all its factors. One of those possible combinations of factors is 3 × 7 × 37 = 777. That is why it is always divisible by that amount.
The total number of dividers
A question that appears in many circumstances, not only in math exercises, but also in our daily lives, has to do with the total number of divisors that a number can have. If it’s a small number, like 6, it’s not too difficult because we do it almost mentally: since 6 = 2 × 3, the divisors are 1, 2, 3 and 6. That is, 4 divisors in all. But what if the number is larger? For example, 32. If we factor 32 we have that it is equal to 2^5. It is also simple, because it only has one prime factor (2). So its divisors are 1, 2, 2^2, 2^3, 2^4, 2^5. That is, there are 6. Can we establish a general rule in these cases? Evidently. If the number factors as p^a, then its divisors are
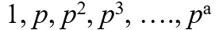
which are just as many as indicated by the exponent (a), plus 1. In short, if we call d(n) the function that provides the number of divisors of the number n, then
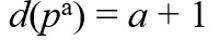
Easy. But things get complicated when the factorization has more than one prime factor, for example, what if n = p^a × q^b? It is also not very complicated using the resource of a table, as follows:
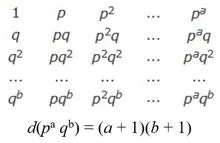
It is easy to deduce that, in general, whatever prime factors we have, the total number of divisors is the product of all the exponents plus one unit in each of them. Then, the total number of divisors provided by the expression above, the one in our enigma, taking into account that 360528 = 2^4 × 3 × 7 × 29 × 37, will be d(360528) = (4+1)(1 +1)(1+1)(1+1)(1+1) = 5 × 2 × 2 × 2 × 2 = 80
Do you dare to find them all?
One final comment on reader responses and the difficulty of the question. I received a couple of perfectly resolved answers by email (from mathematicians or math teachers surely), another was described on twitter, and in the comments of the readers, some also provided the necessary clues for the resolution (via Newton’s binomial, to obtain the coefficients of the division of the polynomials). Others questioned the truth that the Muslim builders of 1492 knew the Gregorian calendar or the exponents in mathematical expressions.
I reiterate once once more that the history in which I framed the exercise is totally invented, but to clarify these questions of the history of mathematics (interesting in themselves, like any other aspect of universal culture), in effect the Gregorian calendar begins to be considered from 1582. But, Arab astronomers and mathematicians had considered other systems to more precisely adjust the length of the years to the movements of the Earth. Specific, Omar Khayyam (see the explanation in this same section) corrected the Persian calendar using the Jalali calendar, which has an error of one day in 3770 years, less than that of the Gregorian calendar, which is one day in 3330 years. The Jalali calendar came into use on March 15, 1079, and is the calendar still used today in countries like Iran and Afghanistan. So although invented by me, the expression might have been written in 1492 by some Muslim mathematician. As for exponents, we also owe Omar Khayyam the earliest description of the power of a binomial with a natural exponent. So the framework in which the question was presented was not so far off the mark. Regarding the difficulty of the question, we might say that it is of medium-high grade for someone who has studied mathematics at some time in their life, and affordable for those who have studied higher mathematics in any university degree.
In any case, my congratulations and thanks to all the readers who tried to solve the question for themselves.

Alfonso Jesús Población Sáez is a professor at the University of Valladolid and a member of the Dissemination Commission of the
Royal Spanish Mathematical Society (RSME).
The ABCdario of Mathematics is a section in collaboration with the RSME.